1. Introduction

Barite (BaSO₄) is a mineral recently found to be practically useful for ESR dating (Okumura et al., 2010; Takamasa et al., 2013; Fujiwara et al., 2015), especially for those occurring in submarine hydrothermal sulfide deposits, although Kasuya et al. (1991) first proposed that dating of barite is possible. Barite crystals are formed when submarine hydrothermal fluid containing Ba is mixed with sea water containing sulfate anions. As the hydrothermal fluid contains large amount of Ra, it incorporates in barite replacing Ba in the crystal lattice. In the hydrothermal sulfide deposits containing barite, most of the natural radiation is from ²²⁶Ra, ²²⁸Ra and their daughter radioactive nuclei where other radioactive elements such as U, Th, and K in sulfide minerals give negligible contribution to the dose rates (Toyoda et al., 2014). When estimating the dose rate to barite crystals, which is a necessary procedure for ESR dating, therefore, internal radiation, especially, that of alpha particles is significant, typically, 40 to 60% of total dose rates (Okumura et al, 2010; Fujiwara et al, 2015).

In the procedure of ESR dating, the age, T, is obtained by

when D is constant, or

when D changes with time. The dose rate, D, is given by

where the dose rate is the sum of doses of alpha, beta, gamma, and cosmic ray dose rates denoted as D_α, D_β, D_γ and D_cos, respectively. It should be noted that, here, the D_E and D are the effective values, that are calibrated by the gamma rays. As alpha particles have a large LET (linear energy transfer), they produce high concentration of electron-hole pairs, hence, higher probability of recombination, i.e., lower effectiveness of trapped unpaired electrons (ESR signal). The ratio, k, is the alpha effectiveness, which is the signal formation rate in the dose response by alpha particles relative to that by gamma rays, has to be used to correct this difference to estimate the “effective” dose rates.

Toyoda et al. (2012) investigated the alpha effectiveness for the ESR signal due to SO₃^- in a barite crystal formed on land, by comparing the dose responses of the signal for gamma irradiation and for He⁺ ion implantation with energy of 4MeV, which simulates the alpha particles. A value 0.043 ± 0.018 was obtained for a sample from Morocco. However, the dose response for He⁺ ion dose is far from “good”. The experiments of He⁺ ion implantation need be repeated to determine the precise alpha effectiveness. In the present paper, we implanted the He⁺ ions accelerated by a Tandem accelerator into two series of barite samples, one synthetic and the other hydrothermal extracted from a sea-floor hydrothermal sulfide deposit. The alpha effectiveness of the SO₃^- is determined as the slope in the dose response to He⁺ ion implantation relative to that to gamma ray irradiation, denoted as

2. Experimental

A natural barite sample was extracted from a hydrothermal deposit taken from the sea-floor at the Hatoma Knoll, and Iheya North Knoll of Okinawa Trough as listed in Table 1 where they were dated by ESR to be 1480 to 6300 years. A block of each sulfide deposit sample was cut into pieces, and about 2.0 g was crushed. The samples were soaked in 12M hydrochloric acid, left for approximately 24 hours. Then, 13M nitric acid was added. Finally, after rinsing in distilled water, the sample was filtered and dried. Impurities were removed by handpicking. An X-ray diffraction study was made to confirm that the grains are pure barite. The samples were further crushed to powder.

Another barite was synthesized by mixing barium chloride (BaCl₂) and sodium sulfate (Na₂SO₄) solutions, as the reaction expressed by

BaCl₂ + NaSO₄ → BaSO₄ + 2NaCl

Aqueous solutions of 300 ml with 0.32 mol/L of barium chloride and of 300 ml with 0.32 mol/L of sodium sulfate, are mixed together at room temperature to have barium sulfate precipitated.

The above powder barite samples of two types (natural and synthetic) were deposited on aluminum plates in deionized water with an area of 2 cm² where the thickness of the barite was about 15 μm (6.75 mg/cm²) while the range of 4 MeV He⁺ ion in barite is about 10 μm (4.5 mg/cm²). The thickness was calculated from the mass difference of the aluminum plates between before and after the deposition. Powder samples (<7 5 μm in grain size) were also prepared for gamma ray irradiation.

A ⁶⁰Co gamma ray source at Takasaki Research Institute of Japanese Atomic Energy Agency (JAEA) was used to irradiate the samples with a dose rate of 467.7 Gy/h to doses up to about 10 kGy.

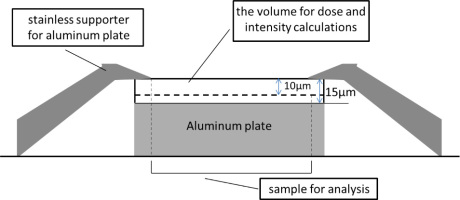
A Tandem accelerator at Takasaki Ion Accelerators for Advanced Radiation Application (TIARA) of Japan Atomic Energy Agency (JAEA) was used to implant the He⁺ ions into the deposited samples where the aluminum plates were attached on a copper plate. The He⁺ ions with an energy of 4 MeV was implanted with a current of about 200 nA in duration of 2 to 80 seconds to an area of about 2 cm². The number of implanted He⁺ ions were monitored by integrating the current during the implantation where the total number ranged 1.2× 10¹² to 5.0×10¹³ ions/cm². The samples were peeled from an aluminum plate for ESR measurements. The doses given to the samples were calculated by dividing the total energy of He⁺ ions, that have passed the surface of the barite samples deposited on an aluminum plates, by the mass of barite sample on the aluminum plate, the surface of which the He⁺ ions passed, and in the volume of which He⁺ ions reached (10 μm from the surface, see Fig. 1).

Fig. 1

The set-up of the experiment for He⁺ ion implantation showing the cross section of the barite sample deposited on an aluminum plate.

After gamma ray irradiation or He⁺ ion implantation, the samples were measured at room temperature with an ESR spectrometer (JES-PX2300) with a microwave power of 1 mW and the magnetic field modulation amplitude of 0.1 mT (Toyoda et al., 2011).

3. Results and Discussions

The ESR spectra observed in the synthetic and a natural hydrothermal (HPD#1358R03) barite samples are shown in Fig. 2. The principal g-values are obtained from this powder spectrum to be 1.9995, 2.0023, and 2.0031, being consistent with the g-values for SO₃^- radical obtained by Ryabov (1983), which are 1.9995, 2.0023, and 2.0032. The shapes of the signals observed in the samples irradiated by gamma rays, those in implanted by He⁺ ions, and that in natural samples are essentially identical.

Fig. 2

The ESR signals observed in (a) a synthetic barite sample and (b) a natural sea-floor hydrothermal barite sample (HPD#1358R03).

The ESR signal intensities were enhanced both by gamma ray irradiation and by He⁺ ion implantation as shown in Fig. 3 where single saturating exponential curves were fitted to the dose responses for natural samples and exponential with linear functions (Duval, 2012) were for the synthetic sample. The scattering of points for He⁺ ion implantation is much smaller than that in Toyoda et al. (2012). The error for the measurement of the signal intensity was typically within 1%, as the standard deviation of the intensities for separate aliquots of a same sample.

Fig. 3

Dose responses of the ESR intensity of SO3^- signal to (a) gamma ray and (b) He⁺ ion implantation doses.

The both dose responses follow the saturating exponential curves (or plus linear), which would indicate that the k values, which are the ratio of the slopes of the tangent on the dose response curves of He⁺ ion implantation and of gamma ray irradiation, may change with dose. The range of the equivalent doses in the actual dating samples is quite low, typically up to about 400 y (e.g. Fujiwara et al., 2015). Therefore, the slope values of the tangents at zero dose are adopted as the formation efficiencies of the signal as the following. For the He⁺ ion dose responses, the inherited natural doses can be neglected as the given doses are much larger (Fig. 3), the slope values of the tangents at given zero dose were adopted. For the gamma ray dose responses, the slope value of the tangent at given zero dose was similarly adopted for the synthetic samples. However, for the young and old natural samples, as the samples have already some given natural doses, the values at the negative equivalent dose values, at which the signal intensity is zero, were adopted as the efficiencies of signal formation by gamma rays, to be used for calculation of the k-values. The obtained efficiency values (the slopes of the tangents) are listed in Table 1. The k values are, then, calculated using the Eq. 1.4 to be 0.053 ± 0.006 for HPD#1621R07 (the young natural sample), to be 0.102 ± 0.022 for HPD#1358R03 (the natural old sample), and to be 0.017 ± 0.001 for the synthetic sample as shown in Table 1.

The obtained k-value is largest in the old natural sample while it is smallest in the synthetic sample. As long as we use natural samples for dating, those for the natural samples should be the candidate. The both efficiency values to He⁺ ion dose and to the gamma ray are smaller in the old natural sample than in the young sample as shown in Table 1 and also in Fig. 3. The old sample has already received substantial amount (D_E = 3240 Gy) of natural beta and gamma and also alpha doses. Therefore, for additional gamma ray dose, it is possible that the sensitive volume in barite, where the ESR signal can be formed, would be less than the young sample, as the part of the volume damaged by the natural alpha particles would be not any more sensitive to the additional gamma ray dose. Although the same mechanism should happen for the volume with natural beta and gamma rays (the volume should be smaller), the latter effect can be considered by fitting a saturating exponential curve, while the former mechanism cannot be simulated by the gamma ray irradiation as the type of the radiation is different. The response to additional gamma ray irradiation would, therefore, be smaller because the sensitive volume, hence mass, is smaller. The same mechanism would be also for the additional He⁺ ion implantation. The additional He⁺ ion implantation will overprint the damage onto the volume with signals created by natural beta and gamma rays, therefore, the response in the older sample would be smaller than in the young sample.

Tentatively, based on the present results, the k-value, 0.053 ± 0.006, obtained for the young natural sample should be adopted for young barite samples (typically up to 1500 years). This value is 19% higher than the previously reported value of 0.043 ± 0.018 (Toyoda et al., 2012). With this value, the ESR ages will typically become about 10% younger than the ones with the old k-values where currently, this difference will not affect the geological interpretations. This k-value would be much more realistic than previous value in the sense that the present value was obtained with using the natural barite extracted from a hydrothermal sulfide deposit which is actually used in dating while Toyoda et al. (2012) examined the barite crystal occurring on land. This value is very similar with the value, 0.052, for calcite speleothem obtained by Lyons and Brennan (1991), and in the same order with those for mollusk shells, 0.07 to 0.10 (Grün, 1985; Grün and Katzenberger, 1994) and with those for corals, 0.05 to 0.15 (Ikeya and Ohmura, 1983; Radtke et al., 1988; Grün et al., 1992; Malmberg and Radtke, 2000).

However, further works would still be needed, firstly, to confirm that the k-value for the present young natural sample, HPD#1621R07, is reproducible in other natural samples. The efficiency value to He⁺ ion dose in the synthetic sample is consistent with the one in the young natural sample as shown in Table 1 while the value to gamma ray dose is higher in the synthetic than in the natural young sample, making the k-value lower. This efficiency value, the saturated intensity value, and the dose value at which saturation starts (typically indicated by D₀) in the dose response would directly be related with the formation mechanism. Indicated by the responses to gamma rays observed in the present study (Fig. 3), it is not so simple as other signal such as one observed in a clay mineral where the higher the saturation value corresponds to the lower the efficiency (Allard and Muller, 1998). In barite, the efficiency to He⁺ ion, hence, alpha dose, is constant while the one to beta and gamma might be sample dependent, which would possibly be due to chemical and physical conditions of barite formation. Secondary, as indicated by the results for the old natural sample, the k-value possibly changes with the alpha dose. It is necessary to examine other samples with variety of ages and also to examine the dose response to the gamma rays in He⁺ ion implanted samples in order to investigate such dependence. If this is the case, some complex numerical calculation with iteration with changing k-value may be necessary.

4. Summary

The value of alpha effectiveness was investigated by the experiments with 4 MeV He⁺ ion implantation and gamma ray irradiation to three barite samples. The obtained dose response of the SO₃^- signal to the He⁺ ion dose is much better than the one previously reported by Toyoda et al. (2012). Tentatively, a value of 0.053 ± 0.006, which was obtained for a young natural barite sample, should be adopted as the k-value. However, the present study also found that the value is sample dependent possibly on the age, therefore, the value should be used for young samples up to 1500 years. Further studies on the k value are still necessary.